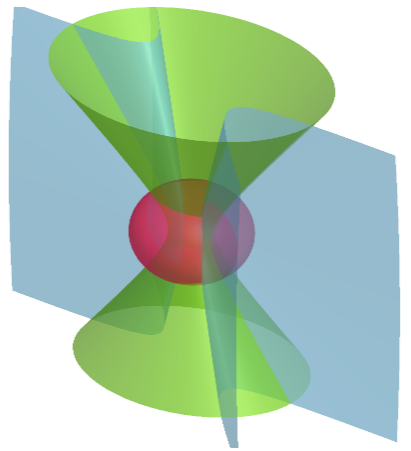
A billiard is a convex domain with a smooth boundary, inside of which we study the trajectory of a ray of light reflecting on the boundary following the law of reflection given by: angle of incidence = angle of reflection. When the trajectory remains tangent to a certain shape, we call this phenomenon caustic (from the Greek καυστός – burnt). Marcel Berger showed that in dimension at least 3, the only billiards in the Euclidean space having caustics are the quadrics. In this talk, we present a result extending Berger’s study to a wider class of billiards called projective billiards, which includes for example billiards in a Minkowski space, in Riemannian manifolds whose geodesics are lines, or in space forms.